Draw Energy Band Diagram for P Type Semiconductor
Extrinsic Semiconductors
- Page ID
- 360
Semiconductors are materials that possess the unique ability to command the menstruum of their charge carriers, making them valuable in applications like prison cell phones, computers, and TVs. An extrinsic semiconductor is a material with impurities introduced into its crystal lattice. The goal of these impurities is to change the electrical backdrop of the material, specifically (increasing) its conductivity. Today, extrinsic semiconductors are a part of innovative, modern engineering devices (including efficient solid state lighting and renewable energy) such equally light emitting diodes, solar cells, lasers, and transistors.
Introduction
Extrinsic semiconductors are merely intrinsic semiconductors that accept been doped with impurity atoms (one dimensional substitutional defects in this case). Doping is the process where semiconductors increase their conductivity by introducing atoms of different elements into their lattice. A semiconductor can be doped past vapor stage epitaxy, where some concentration of impurities in their gaseous phase is contacted with the semiconductor wafer, or by being grown in with the wafer itself with the help of photolithography (microprocessing areas of a wafer), diffusion (gradient controlled particle motion), and ion implantation (utilizing an electrical field to contact an ion with a solid) to increase the dopant concentration in certain parts of the wafer. Based on whether the added impurities are electron donors or acceptors, the semiconductor's Fermi level (the energy state below which all allowable energy states are filled and to a higher place which all states are empty every bit the temperature approaches 0 Kelvin) is able to move either upwards or downwardly from its original position in the center of the energy band gap (the gap between the valence and conduction bands of the semiconductor).
If a semiconducting material is doped with atoms that tin can donate electrons, it is known as an n-type semiconductor which uses those donated electrons to increase its electrical conductivity. If information technology is doped with with atoms that tin accept electrons, it is known as a p-type semiconductor which uses the lack of electrons in the lattice, called holes (tin exist considered as positive charges as well), to increase its conductivity equally well. Aside from being dependent on the concentration of dopants, both due north-type and p-type semiconductors are dependent on temperature changes, especially their electric conductivities, carrier mobilities (how freely a charge carrier tin motility), and even the Fermi levels. The mobilities are based off of 2 different temperature dependent scattering effects, called lattice scattering and ionized impurity scattering. Lattice handful, dominant at higher temperatures, is based on the thermal vibrations of the semiconductor atoms which act every bit obstacles to mobile charge carriers. Ionized impurity scattering, dominant at lower temperatures, depends on the number of dopant ions, which all carry every bit scattering centers, and their ability to negate charge carriers from being moving to dissimilar energy levels because of the electrostatic attraction between ion and carrier known equally Coulomb's Law, described as
\[F = \dfrac{qQ}{4\pi\epsilon_0 r^two} \characterization{1}\]
where q and Q are the charges (contrary charges) on the accuse carrier and dopant ion, \(\epsilon_0\) is the permittivity of free infinite, r is the distance betwixt ion and carrier, and F is the electrostatic bonny force that each of them experience.
No affair what happens with doping, nonetheless, the one equation that always remains true for extrinsic semiconductors is
\[np = n_i ^2 \label{ii}\]
where n is the electron concentration, p is the hole concentration, and\(n_i\) is the intrinsic carrier concentration (the concentration had the semiconductor not been doped).
Northward-type Semiconductors
An n-blazon semiconductor is one that has donor dopants deposited into its crystal lattice. Here, electrons are called the majority carriers and holes are the minority carriers. 1 of the near common examples of this is Silicon or Germanium, from group 4 in the periodic table, being doped with Phosphorus or Arsenic atoms (from grouping V), both of which has one actress valence electron per cantlet. The dopant cantlet is able to enter the lattice, substitute in for one Si atom while bound to four others (a covalent bond), and release its extra, loosely bounded valence electron into the Si lattice.
Every bit stated earlier, the primary reason to inject the semiconducting wafer with impurities is to increase its electrical conductivity, plant to be
\[\sigma = q\mu_n north(T) + q\mu_p p(T) \characterization{one}\]
where q is the accuse of an electron (~ 1.6 *10^-19 Coulombs), \(\mu_n\) and \(\mu_p\) are the electron and pigsty mobilities respectively, and n(T) and p(T) are the concentrations of electrons in the conduction ring or holes in the valence ring. Both the mobilities and the two concentration variables are temperature dependent, notwithstanding for right at present consider the temperature as constant at around room temperature. For all practical purposes in an northward-blazon semiconductor at room temperature, the electrical conductivity term from the pigsty concentration is negligible because commonly, \(N_D\) >> \(n_i\) where \(N_D\) is the concentration of donor dopants, all of whose electrons are in the conduction band at 300 M, and \(n_i\) is the intrinsic carrier concentration of the semiconductor. If the donor electron concentration is much greater than the intrinsic carrier concentration, then the hole concentration contributing to the conductivity is even smaller at \(\dfrac{n_i ^ii}{N_D}\), allowing information technology to be neglected.
Once inserted into the semiconductor, the donor dopants are able to form a donor level in the band gap near the conduction ring, previously where at that place were no existing states, because it is now energetically favorable to do so. This means that the donated electrons will require a much smaller increase in free energy to be excited into the conduction ring, where the free flowing electrons can increase conductivity. Therefore, as doping increases, the conductivity of an north-blazon semiconductor increases besides (more donor states means more donated gratis electrons that can exist promoted into the conduction band).
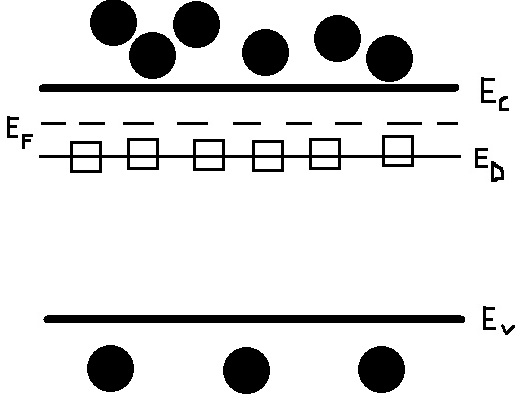
Figure \(\PageIndex{1}\) displays the behavior of the donor doped semiconductor at room temperature. Ec represents the conduction band, Ev is the valence band, \(E_D\) shows the donor level (containing the immobile donor ions), and \(E_F\) is the Fermi level. The blackness circles are electrons, while the white squares correspond the empty, immobile donor states (because all the donor electrons are able to exist promoted into the conduction band at room temperature) that practice not bear upon the conductivity. The electrons beneath Ev are shown, simply to bespeak out that there are however many intrinsic carriers in the valence band that are still yet to be promoted to the conduction ring (a minimal amount of intrinsic have been promoted from the valence band then far, though). At that place is a minimal hole concentration in the valence ring merely this has non been shown in effigy 1, mainly to illustrate the greater importance of electrons in n-blazon semiconductors. Considering that the Fermi level is defined as the states beneath which all allowable energy states are filled and all states above are empty at the temperature approaches 0 Kelvin, information technology makes sense that \(E_F\) would increase from from its heart of the band gap intrinsic position because of the donor level near the conduction ring (filled states are those that are occupied by electrons). Some other mode to think well-nigh this is that the majority of the density of energy states should be independent underneath the Fermi level (at 0 Kelvin, the Fermi level is at the center of the band gap but the donor dopants are not considered equally energy states yet, since all motion ceases). Looking at the equation for Fermi level (ignoring temperature dependence for now since information technology is constant) confirms this, as \[E_F = kTln(\dfrac{N_D}{n_i}) - E_i\]. where \(E_i\) is the is the free energy level in the heart of the band gap. With everything else abiding, increasing the donor concentration increases the Fermi level, significant that electrons can more hands achieve the conduction band.
From this signal, the nigh of import calculation (other than conductivity) that can lead to building efficient devices with multiple applications is to discover the concentration of electrons in the conduction ring. At room temperature, this concentration is but \(N_D\) just in general, it is \[N_C e^\dfrac{E_F - E_C}{kT}\] where \(N_C\) is the density of energy states in the conduction band and k is the Boltzmann constant (1.38 * ten^ -23 Joules/(degree Kelvin)). \(N_C\) can be further simplified equally
\[2[\dfrac{2\pi m_e ^* kT}{h^2}]^\dfrac{3}{2} \label{ii}\]
where \(m_e ^*\) is the effective mass of an electron and h is Planck's abiding (6.626 * 10^-34 J*seconds).
P-type Semiconductors
A p-blazon semiconductor is one that has acceptor dopants deposited into its crystal lattice. Here, holes are called the bulk carriers and electrons are the minority carriers. One of the most common examples of this is Silicon or Germanium, from grouping 4 in the periodic tabular array, being doped with Boron or Gallium atoms (from grouping III), both of which has one fewer valence electron per atom. The dopant cantlet is able to enter the lattice, substitute in for i Si cantlet while spring to 4 others, and take an electron from an adjacent atom into its covalent bond,. This is how the lack of an electron, or hole, moves through the valence band of the material, making it conductive (the same process of replacing a hole with an electron, moving the hole to another location in the lattice keeps repeating).
As mentioned earlier, the primary reason to inject the semiconducting wafer with impurities is to increase its electrical conductivity, which is
\[\sigma = q\mu_n n(T) + q\mu_p p(T) \label{3}\]
where q is the charge of an electron, \(\mu_n\) and \(\mu_p\) are the electron and hole mobilities respectively, and n(T) and p(T) are the concentrations of electrons in the conduction band or holes in the valence band. Both the mobilities and the two concentration variables are temperature dependent, however for right now consider the temperature as constant at around room temperature. For all practical purposes in a p-type semiconductor at room temperature, the conductivity term from the electron concentration is negligible because usually, \(N_A\) >> \(n_i\) where \(N_A\) is the concentration of acceptor dopants, all of whose holes are in the valence band by 300 1000, and \(n_i\) is the intrinsic carrier concentration of the semiconductor. If the hole concentration is much greater than the intrinsic carrier concentration, then the electron concentration contributing to the conductivity is even smaller at \(\dfrac{n_i ^ii}{N_A}\), allowing it to exist neglected.
Once inserted into the semiconductor, the acceptor dopants are able to form an acceptor level in the ring gap near the valence band, previously where there were no existing states, because information technology is now energetically favorable to practice so. This ways that the electrons in the valence band can be excited into this acceptor level in the band gap (every bit opposed to the conduction band) to complete the covalent bond. This process leaves backside free holes that are able to propagate through the valence band, where they can increment the conductivity. Therefore, as doping increases, the conductivity of a p-blazon semiconductor as well increases (more than acceptor states means more complimentary holes that can permeate the valence ring).
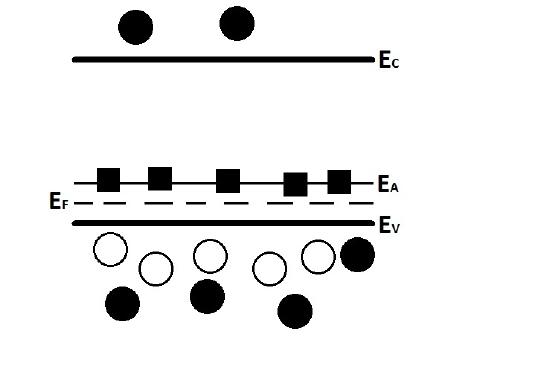
Figure 2 displays the behavior of the acceptor doped semiconductor at room temperature. Ec represents the conduction band, Ev is the valence band, \(E_A\) shows the acceptor level (containing the immobile acceptor ions), and \(E_F\) is the Fermi level. The black circles are electrons, while the blackness squares represent the empty (electron rich but devoid of holes), immobile acceptor states (because all the acceptor ions' holes are able to pervade the valence ring at room temperature) that do not touch the conductivity. The white circles are the holes that that have formed due to the acceptor dopants requiring electrons to complete their covalent bonding. At that place are more than holes than electrons in the valence band in the motion picture because holes are the majority carriers. The electrons higher up Ev are shown, just to point out that in that location are carriers that that exist in the conduction band that accept been promoted from the valence ring (a minimal corporeality have been promoted from the valence ring, though). Because that the Fermi level is divers as united states of america below which all allowable energy states are filled and all states above are empty at the temperature approaches 0 Kelvin, it makes sense that \(E_F\) would decrease from from its middle of the ring gap intrinsic position because of the acceptor level almost the valence ring ("filled" states are occupied by electrons). Another way to retrieve about this is that the bulk of the density of energy states should exist contained underneath the Fermi level (at 0 Kelvin, the Fermi level is at the middle of the band gap and the acceptor dopants are not considered every bit free energy states anyways since all motion ceases and at that place are no mobile electrons present still, simply holes) The downward shift of the Fermi level highlights the fact that p-type semiconductors emphasizes conduction of holes in the valence band as opposed to conduction of electrons in the conduction band, which means that the number of electrons above \(E_F\) are at a minimum. Looking at the equation for Fermi level (ignoring temperature dependence for now since it is constant) confirms this, equally
\[E_F = -kT\ ln \left(\dfrac{N_D}{n_i} \right) + E_i. \label{4}\]
where \(E_i\) is the is the energy level in the eye of the band gap. With everything else constant, increasing the acceptor concentration decreases the Fermi level, pregnant that holes can more hands reach the valence ring.
From this point, the most important adding (other than conductivity) that tin can lead to creating innovative, versatile devices is to find the concentration of holes in the valence ring. At room temperature, this concentration is simply \(N_A\) just in general, it is
\[N_V e^\dfrac{E_V - E_F}{kT} \characterization{5}\]
where \(N_V\) is the density of energy states in the valence band and thousand is the Boltzmann constant. \(N_C\) tin can be further simplified every bit \[2[\dfrac{two\pi m_h^* kT}{h^2}]^\dfrac{three}{ii}\] where \(m_h ^*\) is the effective mass of a pigsty and h is Planck's abiding.
Temperature Dependence
Earlier exploring the temperature dependence of electrical conductivities, carrier mobilities (with lattice and ionized impurity handful), and Fermi levels, the doping dependence of ionized impurity scattering needs to be considered.
In general, scattering decreases electron mobility of charge carriers past acting as obstacles. In other words, accuse carriers are non able to menses easily throughout the textile. Ionized impurity scattering occurs when at that place are many donor or acceptor dopants present in the lattice. As doping increases, each added donor or acceptor level tin can be thought of equally an additional "scattering center" added to the lattice, another obstruction that tin impede charge carrier motion. This type of scattering does not depend on any sort of thermal vibration, just rather takes reward of the fact that carriers do non have enough energy to move to any different energy levels. This ways that for northward-type semiconductors, the ionized donor atoms, positive ions, are able to crusade an electrostatic attraction with the donated electrons while in p-type semiconductors, the ionized acceptor atoms (negative ions) crusade an electrostatic attraction with the holes created in the lattice. Carrier Free energy barriers are not an issue when thermal energy is a major factor. This means that for both due north and p-type conductors, ionized impurity scattering is ascendant at lower temperatures and its effects gradually decrease as the temperature increases.
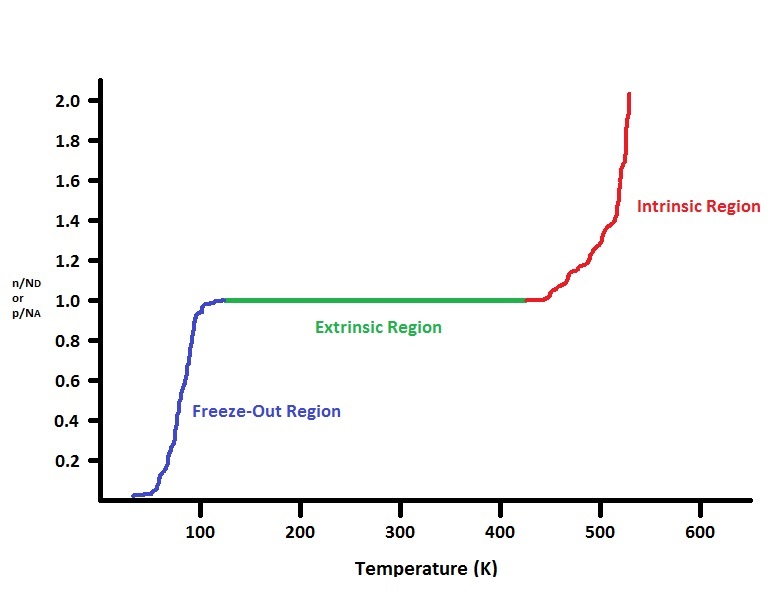
Figure three clearly demonstrates the phases an extrinsic semiconductor's majority carrier goes through while existence heated upward while property the corporeality of dopants constant. For an n-type semiconductor, the beginning of the Freeze-Out Region represents the expanse where the donor ions are situated at the donor level, simply the complimentary electrons are unable to move due to the 0 Kelvin temperature. Equally the temperature increases in the blue government, a few of the donor electrons go excited into the conduction band while others are even so impeded due to the ionized impurity scattering, which decreases the overall mobility of the electrons. As the temperature starts increasing toward the Extrinsic Region, the result of ionized impurity scattering slowly fades abroad, increasing the electron mobility, and the amount of electrons being promoted from the donor level to the conduction band increases even more than. At this point, a minimal corporeality of the intrinsic electrons are promoted from the valence band (however this is still considered as a negligible issue). Since conductivity directly depends on electron mobility and the concentration of electrons in the conduction band, which increases with increasing temperature co-ordinate to the equation near the stop of the N-Type Semiconductor section, the electrical conductivity too increases. Throughout the green regime, all the donor level electrons are excited into the conduction band and neither handful event is prominent, which means that the conductivity increases even more. Meanwhile, a few more intrinsic electrons from the valence band are promoted into the conduction ring, even so this is still negligible compared to the concentration of donated electrons already in or above \(E_V\). As the temperature proceeds into the Intrinsic Region, intrinsic electrons from the valence band are excited into the conduction band at a faster charge per unit (leaving backside an equivalent amount of holes in the valence band) and the conductivity continues to increment. All the same, this is when the lattice handful mechanism is activated. This means that the semiconductors atoms in the lattice undergo thermal vibrations, which act equally obstacles to the moving electrons, causing the mobility to decrease (since the electron concentration is more than important, conductivity still increases fifty-fifty as mobility begins to decrease). Throughout the scarlet regime, the electrons from the valence band are promoted into the conduction band and create holes in the valence ring. Equally this continues, the north-type semiconductor essentially becomes an intrinsic semiconductor, with all the intrinsic electrons at present in the conduction ring and the equivalent amount of holes left behind in the valence band. Lattice scattering is at its greatest due to the thermal vibrations, significant that the electron mobility decreases even further. However, conductivity is at its greatest because even though the electrons have a harder time moving through the material, there are and so many more of them at higher temperatures.
In this entire procedure, the most complex concept to understand is the Fermi level, which is best decribed in words and not equations for temperature changes. In the get-go blue region, the Fermi level is notwithstanding at the middle of the band gap considering all move stops and there are no densities of states that even exist. Through the blue region towards the point where the material acts like an northward-type semiconductor, the Fermi level moves up to a bespeak where the majority of the states are contained underneath it (Fermi level between conduction ring and donor level). However, every bit more than intrinsic electrons commencement adding to the electrical conductivity in the red regime of the figure, the Fermi level starts moving down closer to the middle of the ring gap resembling an intrinsic semiconductor.
For p-type semiconductors, the conductivity and mobility trends follow those of the n-blazon semiconductor along figure iii. Equally temperature increases, ionized impurity scattering furnishings subtract (increasing mobility) because holes gain enough energy to move towards the valence ring and electrons from the valence band occupy the acceptor states to complete the covalent bonds, which increases the holes' electrical conductivity. The hole mobility increases fifty-fifty more at the Extrinsic Region because ionized impurity scattering fades abroad and lattice scattering has not been activated yet. Meanwhile, all the acceptor states are devoid of holes, which means that the conductivity throughout the valence band increases even more than. At this bespeak, there are a few intrinsic electrons (nonetheless a negligible effect) that are excited into the conduction ring. Equally the temperature increases into the Intrinsic Region, more intrinsic electrons are promoted into the conduction band, but nonetheless once again the lattice scattering mechanism is activated, where the semiconductor atoms brainstorm the vibrate due to increased thermal energy, this impeded the motion of all the accuse carriers, causing both mobilities to decrease. Withal, since electrical conductivity is more than heavily determined by the carrier concentrations (which increment with increasing temperature) information technology continues to increment. Through the red authorities, more intrinsic electrons in the valence band get excited into the conduction band at a faster charge per unit, leaving behind more holes. As this continues, the p-type semiconductor gradually becomes an intrinsic semiconductor with all the intrinsic electrons that are promoted, leaving backside even more holes. The lattice scattering is at its highest point from all the thermal vibrations (electron and hole mobilities decrease even further). However, conductivity is at its maximum point because fifty-fifty though both charge carriers experience multiple scattering events, there are then many more than holes and electrons that conduct in their respective bands at higher temperatures.
Once again through this process, the most complex concept to understand is the Fermi level, (best decribed verbally and not mathematically for temperature changes). In the beginning blue region, the Fermi level is even so at the middle of the ring gap because all motion stops and there are no densities of states that fifty-fifty exist. Through the blue region towards the indicate where the material acts like an p-type semiconductor, the Fermi level moves downwardly to a point where the bulk of the states are contained underneath it (Fermi level between valence band and acceptor level). Even so, as more than intrinsic electrons outset adding to the conductivity in the ruby-red regime of the effigy, the Fermi level starts moving up closer to the middle of the band gap resembling an intrinsic semiconductor.
Questions
- What is the primary difference between an n-type and a p-blazon semiconductor?
- Proper name and draw the departure between the two unlike types of scattering events.
- What are the three temperature dependent regions/behaviors for an extrinsic semiconductor?
Answers
- An northward-type semiconductor has been doped with impurity atoms that contains one more electron per cantlet, assuasive them to donate electrons which can bear in the conduction band. A p-type semiconductor has been doped with impurity atoms that contain ane fewer electron per cantlet, letting them accept electrons and behave a "lack" of electrons, or holes, in the valence band.
- Lattice scattering occurs at higher temperatures because information technology deals with activating thermal vibrations that impede charge carrier motility which decreases the overall carrier mobility. As temperature decreases, thermal vibrations are reduced and lattice handful loses its result. Lattice scattering has no measurable doping dependence. Ionized impurity scattering occurs at low temperatures and increases with increasing dopants. This type of scattering machinery takes reward of the fact that at low temperatures, carriers do not have enough energy to move to whatsoever dissimilar energy levels, meaning that the ionized dopant ions cause an electrostatic allure with electrons or holes in an due north-type or p-type semiconductor respectively. Increasing the number of dopants adds to the number of "scattering centers" in a semiconducting material, meaning more obstacles that can limit the movement of charge carriers.
- The 3 regions/behaviors are the: Freeze-Out Region, Extrinsic Region, and Intrinsic Region.
References
- R Pierret. (1996). Semiconductor Device Fundamentals. Reading, Mass.: Addison-Wesley, 1996. 29-38. Print.
- R Hummel. (2012). Electronic Properties of Materials (4th ed.). New York, NY: Springer, 2012. Impress.
- South Kasap and P Capper. (2007). Springer Handbook of Electronic and Photonic Materials (4th ed.).
Contributors and Attributions
- Niteesh Marathe (University of California, Davis Grade of 2015)
Source: https://eng.libretexts.org/Bookshelves/Materials_Science/Supplemental_Modules_(Materials_Science)/Semiconductors/Extrinsic_Semiconductors
0 Response to "Draw Energy Band Diagram for P Type Semiconductor"
Post a Comment